 |
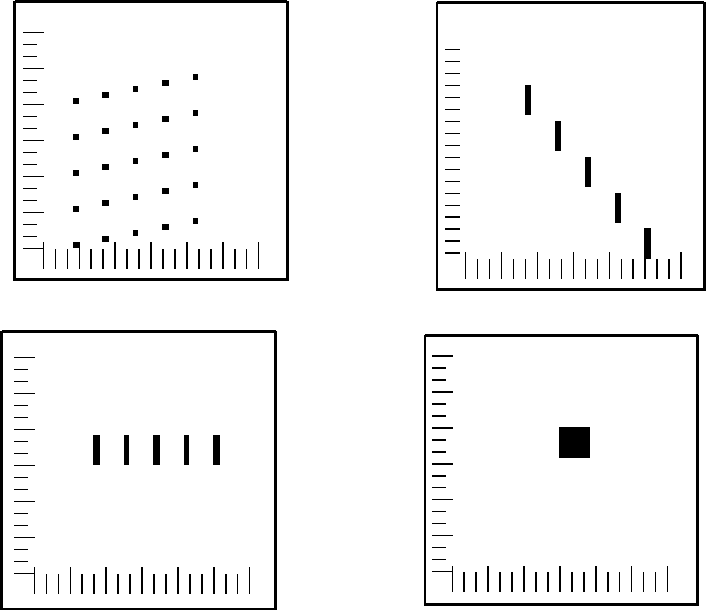
The next case is that of a four-dimensional hyperplane extending across dimension 2 and diagonally through the other three dimensions. This example is significant in that standard projection techniques often fail to convey this sort of cross-dimensional structure. Figure 4a shows the display of the hyperplane when first read into N-Land. While there does seem to be a pattern to the data, it is not completely clear what it actually is.
The first step is to permute the speeds for that dimensional channel, which brings out some visible structure as seen in Figure 4b. Viewed in this manner, the orientation of the hyperplane begins to become apparent. A positive 45 degree shear is applied with dimension 3 as the shearing axis and the dimension 4 as the fixed axis. The result can be seen in Figure 4c. Finally, another positive shear of 45 degrees is applied with dimension 1 as the fixed axis and dimension 3 as the shearing axis. This should have caused the data to appear as a plane, but does not. By flipping through the channels, the plane indeed becomes visible, as seen in Figure 4d.
 |